Nonagon - Displaying top 8 worksheets found for this concept. Some of the worksheets for this concept are 6 introduction to polygons, Shapes octagon 10 decagon heptagon, 6 polygons and angles, Triangle heptagon hexagon decagon, Define and identify polygons, Geo hw, Work 1 revised convex polygons.
These cards are all about Shapes . These bingo cards include words like you can create cards like these using BingoCardCreator
How To Play Shapes Bingo Cards
- Print and go Shapes, Customize or start from scratch with Shapes bingo cards by logging in or registering your Bingo Card Creator
- Give one card to each player.
- call off words randomly from the cut-aprt call list automatically genarated when you print your Shapes. You can either just call out the word or give more involved clue where the answer is.
- Players mark the word on their card.
- Get the prize bag ready! The first player to mark words in an entire row, column or diagonal wins!.
Timing Tips:
How long a bingo game lasts depends on what pace you read the clues at and how many players you have. If you read faster, such as for older or more experienced students, or if you have more players, the game tends to end more quickly. In general, I suggest allocating between twenty and thirty minutes to a bingo game. Since they can potentially end as early as the fourth word called, though that is quite rare, I encourage you to keep playing in the event of the game being over earlier than you expected it to be. Almost 90% of bingo games with a 25 word list and 25 players will see their first bingo within 7 to 11 words being called.
Card Customization Tip:
You can use Bingo Card Creator to change the card size. This will help if you do not have a lot of time or for younger audiences.
You can use Bingo Card Creator to add pictures or change the free space to make a truly personalized bingo game .
Words List For shapes Bingo Cards
- triangle
- trapezoid
- square
- sphere
- sector
- rhombus
- rectangle
- pyramid
- polygon
- poly
- pentagon
- oval
- octagon
- nonagon
- line
- line
- hexagon
- heptagon
- dodecagon
- decagon
- cylinder
- cube
- crescent
- cone
- circle
- arc
| Regular enneagon (nonagon) | |
|---|---|
| Type | Regular polygon |
| Edges and vertices | 9 |
| Schläfli symbol | {9} |
| Coxeter diagram | |
| Symmetry group | Dihedral (D9), order 2×9 |
| Internal angle (degrees) | 140° |
| Dual polygon | Self |
| Properties | Convex, cyclic, equilateral, isogonal, isotoxal |
In geometry, a nonagon (/ˈnɒnəɡɒn/) or enneagon (/ˈɛniəɡɒn/) is a nine-sided polygon or 9-gon.
The name nonagon is a prefixhybrid formation, from Latin (nonus, 'ninth' + gonon), used equivalently, attested already in the 16th century in French nonogone and in English from the 17th century. The name enneagon comes from Greekenneagonon (εννεα, 'nine' + γωνον (from γωνία = 'corner')), and is arguably more correct,[1] though less common than 'nonagon'.
Regular nonagon[edit]
A regular nonagonPing my airpods. is represented by Schläfli symbol {9} and has internal angles of 140°. The area of a regular nonagon of side length a is given by
where the radius r of the inscribed circle of the regular nonagon is
and where R is the radius of its circumscribed circle:
Construction[edit]
Although a regular nonagon is not constructible with compass and straightedge (as 9 = 32, which is not a product of distinct Fermat primes), there are very old methods of construction that produce very close approximations.[2]
It can be also constructed using neusis, or by allowing the use of an angle trisector.
Super mario maker bookmark profile.
The nonagon can be constructed with 36 meccano equal bars. The construction includes 9 equilateral triangles. Bars are disposed in four levels. Physical models are possible selecting correctly the size of the pieces in order bars and bolts don't obstruct anything else.
Approximations[edit]
Approximation I[edit]
- Accuracy (linear): 10−6
The following is an approximate construction of a nonagon using a straightedge and compass.
Example to illustrate the error, when the constructed central angle is 39.99906°:
At a circumscribed circle radius r = 100 m, the absolute error of the 1st side would be approximately 1.6 mm.
Approximation II[edit]
- Accuracy (linear): 10−10
- Downsize the angle JMK (also 60°) with four bisections of angle and make a thirds of circular arc MON with an approximate solution between bisections of angle w3 and w4.
- Straight auxiliary line g aims over the point O to the point N (virtually a ruler at the points O and N applied), between O and N, therefore no auxiliary line.
- Thus, the circular arc MON is freely accessible for the later intersection point R.
- RMK = 40.0000000052441..°
- 360° ÷ 9 = 40°
- RMK - 40° = 5.2..E-9°
- Example to illustrate the error:
- At a circumscribed circle radius
- r = 100,000 km, the absolute error of the 1st side would be approximately 8.6 mm.
See also the calculation (Berechnung, German).
Nonagon at a given circumscribed circle, animation]]
Nonagon at a given side length, animation
Symmetry[edit]
The regular enneagon has Dih9 symmetry, order 18. There are 2 subgroup dihedral symmetries: Dih3 and Dih1, and 3 cyclic group symmetries: Z9, Z3, and Z1.
These 6 symmetries can be seen in 6 distinct symmetries on the enneagon. John Conway labels these by a letter and group order.[4] Full symmetry of the regular form is r18 and no symmetry is labeled a1. The dihedral symmetries are divided depending on whether they pass through vertices (d for diagonal) or edges (p for perpendiculars), and i when reflection lines path through both edges and vertices. Cyclic symmetries in the middle column are labeled as g for their central gyration orders.
Each subgroup symmetry allows one or more degrees of freedom for irregular forms. Only the g9 subgroup has no degrees of freedom but can seen as directed edges.
Tilings[edit]
The regular enneagon can tessellate the euclidean tiling with gaps. These gaps can be filled with regular hexagons and isosceles triangles. In the notation of symmetrohedron this tiling is called H(*;3;*;[2]) with H representing *632 hexagonal symmetry in the plane.
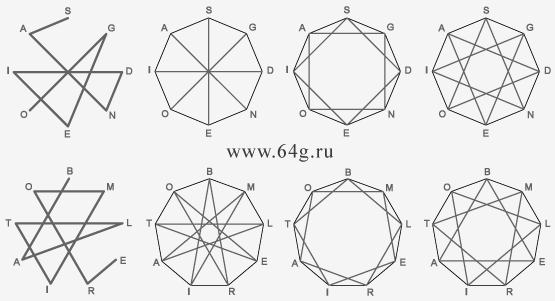
Graphs[edit]

The K9complete graph is often drawn as a regular enneagon with all 36 edges connected. This graph also represents an orthographic projection of the 9 vertices and 36 edges of the 8-simplex.
8-simplex (8D) |
Pop culture references[edit]
- They Might Be Giants have a song entitled 'Nonagon' on their children's album Here Come the 123s. It refers to both an attendee at a party at which 'everybody in the party is a many-sided polygon' and a dance they perform at this party.[5]
- Slipknot's logo is also a version of a nonagon, being a nine-pointed star made of three triangles, referring to the nine members.
- King Gizzard & the Lizard Wizard have an album titled 'Nonagon Infinity', the album art featuring a nonagonal complete graph. The album consists of nine songs and repeats cyclically.
Architecture[edit]
Temples of the Bahá'í Faith, called Bahá'í Houses of Worship, are required to be nonagonal.
The U.S. Steel Tower is an irregular nonagon.
See also[edit]
- Enneagram (nonagram)
References[edit]
- ^Eric W. Weisstein. 'Nonagon'. > MathWorld--A Wolfram Web Resource. Retrieved 24 October 2018.
- ^J. L. Berggren, 'Episodes in the Mathematics of Medieval Islam', p. 82 - 85 Springer-Verlag New York, Inc. 1st edition 1986, retrieved on 11 December 2015.
- ^Ernst Bindel, Helmut von Kügelgen. 'KLASSISCHE PROBLEME DES GRIECHISCHENALTERTUMS IM MATHEMATIKUNTERRICHT DER OBERSTUFE'(PDF). ERZIEHUNGSKUNST. Bund der Freien Waldorfschulen Deutschlands. pp. 234–237.Retrieved on 14 July 2019.
- ^John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, (2008) The Symmetries of Things, ISBN978-1-56881-220-5 (Chapter 20, Generalized Schaefli symbols, Types of symmetry of a polygon pp. 275-278)
- ^TMBW.net
External links[edit]
- Properties of a Nonagon (with interactive animation)